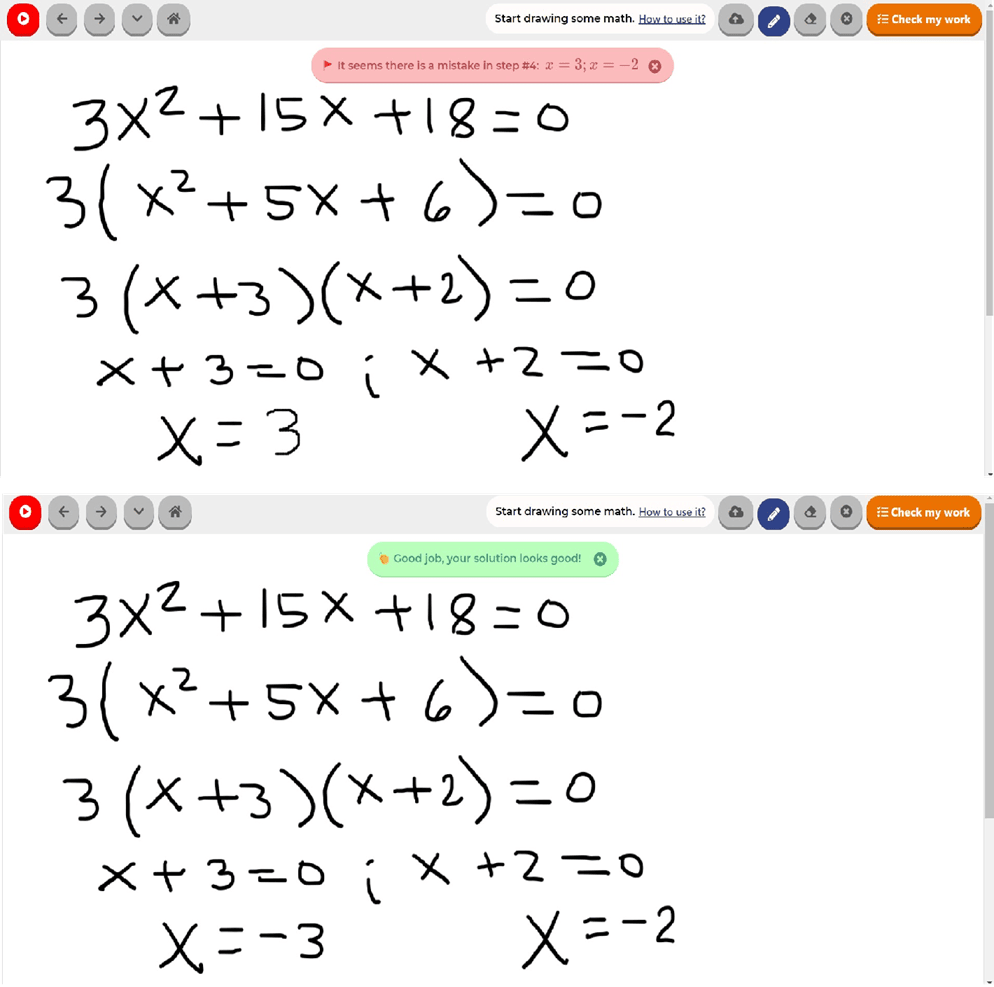
Réponse finale au problème
Solution étape par étape
Comment résoudre ce problème ?
- Choisir une option
- Écrire sous la forme la plus simple
- Simplifier
- Facteur
- Trouver les racines
- En savoir plus...
Diviser $x^6$ par $x^2+1$
Apprenez en ligne à résoudre des problèmes simplification des fractions algébriques étape par étape.
$\begin{array}{l}\phantom{\phantom{;}x^{2}+1;}{\phantom{;}x^{4}\phantom{-;x^n}-x^{2}\phantom{-;x^n}+1\phantom{;}\phantom{;}}\\\phantom{;}x^{2}+1\overline{\smash{)}\phantom{;}x^{6}\phantom{-;x^n}\phantom{-;x^n}\phantom{-;x^n}\phantom{-;x^n}\phantom{-;x^n}\phantom{-;x^n}}\\\phantom{\phantom{;}x^{2}+1;}\underline{-x^{6}\phantom{-;x^n}-x^{4}\phantom{-;x^n}\phantom{-;x^n}\phantom{-;x^n}\phantom{-;x^n}}\\\phantom{-x^{6}-x^{4};}-x^{4}\phantom{-;x^n}\phantom{-;x^n}\phantom{-;x^n}\phantom{-;x^n}\\\phantom{\phantom{;}x^{2}+1-;x^n;}\underline{\phantom{;}x^{4}\phantom{-;x^n}+x^{2}\phantom{-;x^n}\phantom{-;x^n}}\\\phantom{;\phantom{;}x^{4}+x^{2}-;x^n;}\phantom{;}x^{2}\phantom{-;x^n}\phantom{-;x^n}\\\phantom{\phantom{;}x^{2}+1-;x^n-;x^n;}\underline{-x^{2}\phantom{-;x^n}-1\phantom{;}\phantom{;}}\\\phantom{;;-x^{2}-1\phantom{;}\phantom{;}-;x^n-;x^n;}-1\phantom{;}\phantom{;}\\\end{array}$
Apprenez en ligne à résoudre des problèmes simplification des fractions algébriques étape par étape. (x^6)/(x^2+1). Diviser x^6 par x^2+1. Polynôme résultant.