Final answer to the problem
$y=\frac{1}{2}\ln\left(\frac{1+\sin\left(x\right)}{1-\sin\left(x\right)}\right)$
Got another answer? Verify it here!
Step-by-step Solution
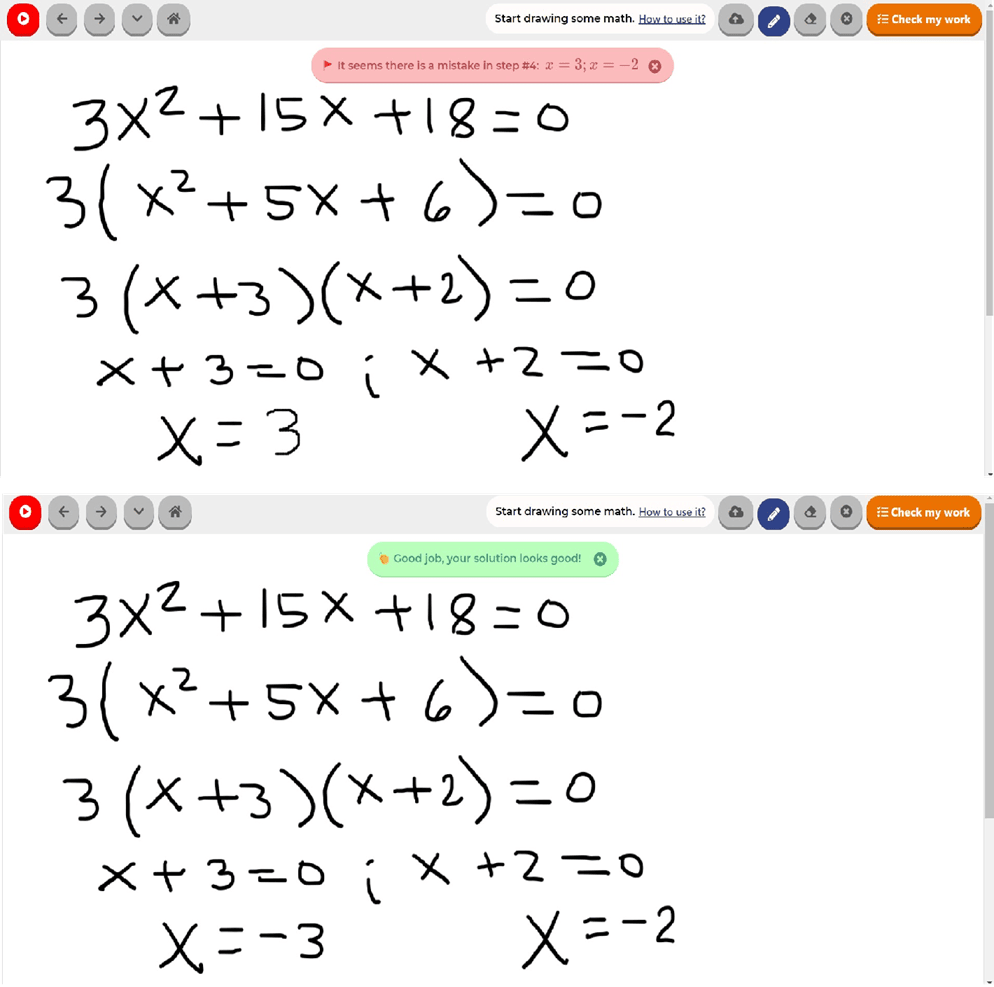
How should I solve this problem?
- Choisir une option
- Differential
- Simplifier
- Facteur
- Load more...
Can't find a method? Tell us so we can add it.
1
Apply the formula: $\ln\left(x^a\right)$$=a\ln\left(x\right)$, where $a=\frac{1}{2}$ and $x=\frac{1+\sin\left(x\right)}{1-\sin\left(x\right)}$
$y=\frac{1}{2}\ln\left(\frac{1+\sin\left(x\right)}{1-\sin\left(x\right)}\right)$
Final answer to the problem
$y=\frac{1}{2}\ln\left(\frac{1+\sin\left(x\right)}{1-\sin\left(x\right)}\right)$