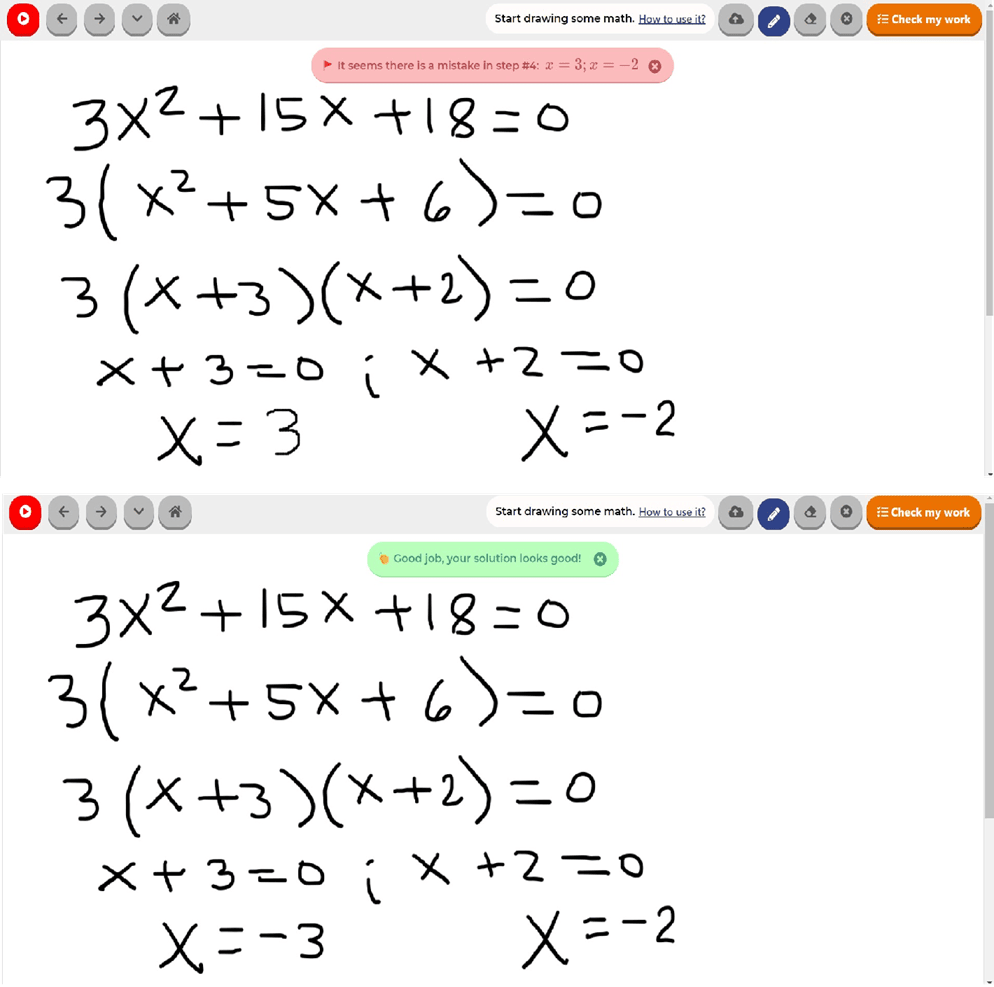
Réponse finale au problème
Solution étape par étape
Comment résoudre ce problème ?
- Choisir une option
- Produit de binômes avec terme commun
- Méthode FOIL
- En savoir plus...
Appliquer la formule : $\frac{d}{dx}\left(a^b\right)$$=y=a^b$, où $d/dx=\frac{d}{dx}$, $a=\mathrm{tanh}\left(2x\right)$, $b=x$, $a^b=\mathrm{tanh}\left(2x\right)^x$ et $d/dx?a^b=\frac{d}{dx}\left(\mathrm{tanh}\left(2x\right)^x\right)$
Apprenez en ligne à résoudre des problèmes étape par étape.
$y=\mathrm{tanh}\left(2x\right)^x$
Apprenez en ligne à résoudre des problèmes étape par étape. d/dx(tanh(2x)^x). Appliquer la formule : \frac{d}{dx}\left(a^b\right)=y=a^b, où d/dx=\frac{d}{dx}, a=\mathrm{tanh}\left(2x\right), b=x, a^b=\mathrm{tanh}\left(2x\right)^x et d/dx?a^b=\frac{d}{dx}\left(\mathrm{tanh}\left(2x\right)^x\right). Appliquer la formule : y=a^b\to \ln\left(y\right)=\ln\left(a^b\right), où a=\mathrm{tanh}\left(2x\right) et b=x. Appliquer la formule : \ln\left(x^a\right)=a\ln\left(x\right), où a=x et x=\mathrm{tanh}\left(2x\right). Appliquer la formule : \ln\left(y\right)=x\to \frac{d}{dx}\left(\ln\left(y\right)\right)=\frac{d}{dx}\left(x\right), où x=x\ln\left(\mathrm{tanh}\left(2x\right)\right).