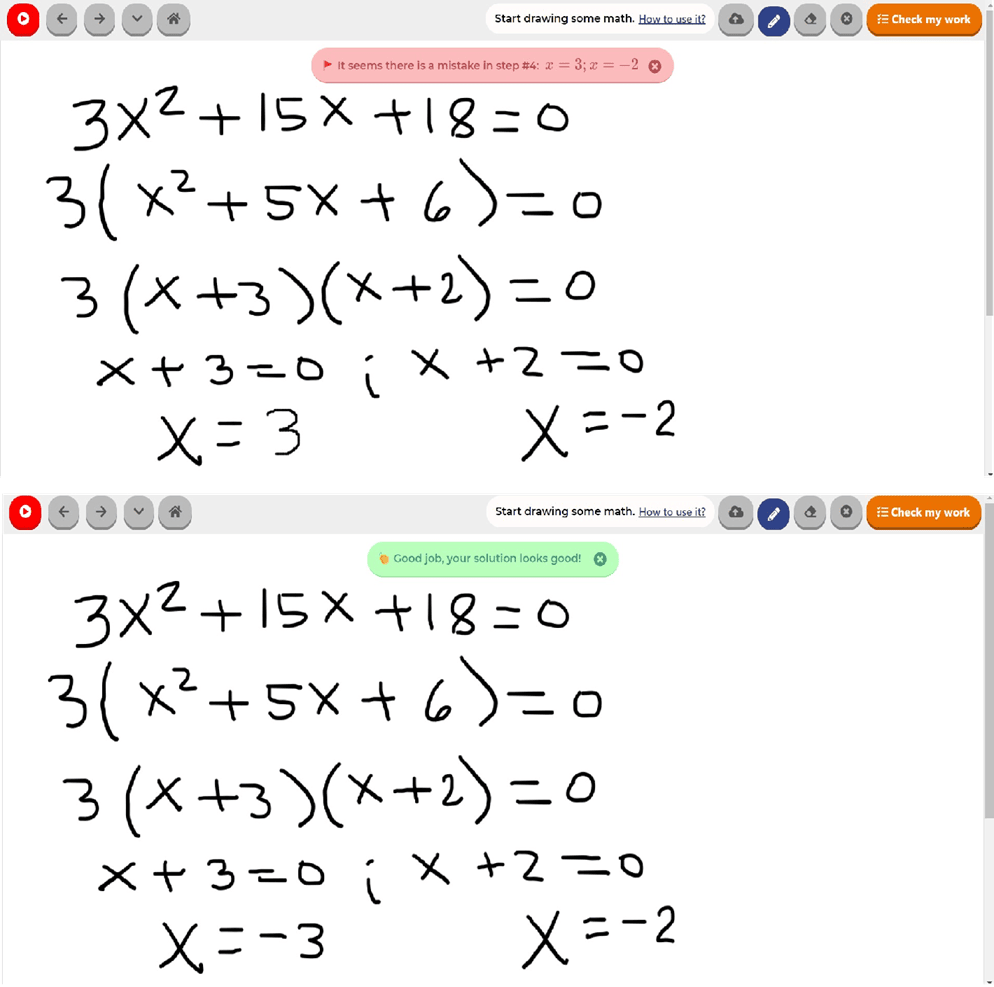
Final answer to the problem
Step-by-step Solution
How should I solve this problem?
- Choisir une option
- Produit de binômes avec terme commun
- Méthode FOIL
- Load more...
Apply the formula: $\frac{d}{dx}\left(x\right)$$=y=x$, where $d/dx=\frac{d}{dx}$, $d/dx?x=\frac{d}{dx}\left(\frac{\sqrt{x+2}-\sqrt{2}}{x}\right)$ and $x=\frac{\sqrt{x+2}-\sqrt{2}}{x}$
Learn how to solve problems step by step online.
$y=\frac{\sqrt{x+2}-\sqrt{2}}{x}$
Learn how to solve problems step by step online. Find the derivative d/dx(((x+2)^(1/2)-2^(1/2))/x). Apply the formula: \frac{d}{dx}\left(x\right)=y=x, where d/dx=\frac{d}{dx}, d/dx?x=\frac{d}{dx}\left(\frac{\sqrt{x+2}-\sqrt{2}}{x}\right) and x=\frac{\sqrt{x+2}-\sqrt{2}}{x}. Apply the formula: y=x\to \ln\left(y\right)=\ln\left(x\right), where x=\frac{\sqrt{x+2}-\sqrt{2}}{x}. Apply the formula: y=x\to y=x, where x=\ln\left(\frac{\sqrt{x+2}-\sqrt{2}}{x}\right) and y=\ln\left(y\right). Apply the formula: \ln\left(y\right)=x\to \frac{d}{dx}\left(\ln\left(y\right)\right)=\frac{d}{dx}\left(x\right), where x=\ln\left(\sqrt{x+2}-\sqrt{2}\right)-\ln\left(x\right).