Créez de beaux diagrammes qui mettent en évidence ce qui compte le plus


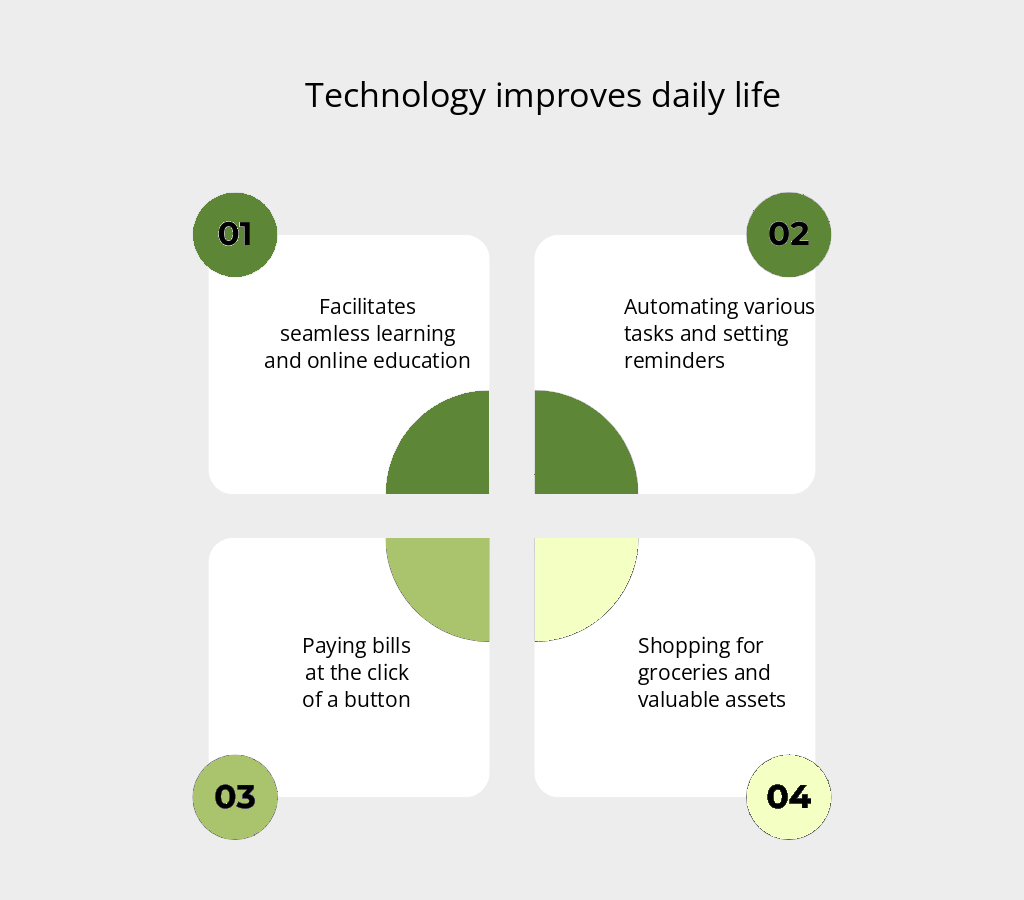






























Nous savons que les mathématiques peuvent être difficiles, cest pourquoi nous sommes là pour vous aider. SnapXam est un tuteur mathématique alimenté par lIA, qui vous aidera à comprendre comment résoudre les problèmes de mathématiques, de larithmétique au calcul. Gagnez du temps en comprenant les concepts mathématiques et en trouvant des vidéos explicatives.
Avec SnapXam, passer des heures et des heures à étudier pour essayer de comprendre appartient au passé. Apprenez à résoudre les problèmes dune meilleure façon et en une fraction du temps.
Sinscrire gratuitementProblèmes résolus
5M+
Lapprentissage des élèves
500k+
dans plus de 100 pays

Accédez aux solutions à vos problèmes depuis tous vos appareils, à tout moment.
Avoir SnapXam, cest comme avoir un livre avec des milliers de problèmes résolus, qui tient dans la poche !
Voir nos calculatrices